➔ Calculate Stiffness for Semi-Elliptic Laminated Leaf Spring
➔ Calculate Stiffness for Quarter-Elliptic Laminated Leaf Spring
Leaf Springs Calculator
To calculate the stiffness of laminated leaf springs, you need to use specific formulas based on the type of spring. Here's how to calculate the stiffness for both semi-elliptic and quarter-elliptic laminated leaf springs:
1. Semi-Elliptic Laminated Leaf Spring Stiffness Calculation
For a semi-elliptic laminated leaf spring, the stiffness can be calculated using the following formula:
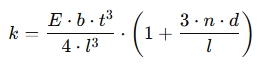
Where:
- = Spring stiffness (N/m or lb/in)
- = Modulus of elasticity of the material (Pa or psi)
- = Width of the leaf spring (m or inches)
- = Thickness of the leaf spring (m or inches)
- = Length of the leaf spring (m or inches)
- = Number of leaves in the spring
- = Distance between the center of the spring and the point of load application (m or inches)
2. Quarter-Elliptic Laminated Leaf Spring Stiffness Calculation
For a quarter-elliptic laminated leaf spring, the stiffness can be calculated using a slightly different formula:

Where the variables are the same as the previous formula, but the length ll and the number of leaves nn may have slightly different impact depending on the specific design of the spring.