Shortest distance between two lines calculator
| The straight line passes through A(a1,b1,c1) and is parallel to the vector V1(p1,q1,r1) |
| Point A | ( | , | , | ) | |||
| Vector V1 | ( | , | , | ) |
| The straight line passes through B(a2,b2,c2) and is parallel to the vector V2(p2,q2,r2) |
| Point B | ( | , | , | ) | |||
| Vector V2 | ( | , | , | ) |
| The shortest distance between two straight lines(d) |
First, transform the equation of the line into a symmetrical form to obtain its direction vector n1=(a1,b1,c1),n2=(a2,b2,c2).
Multiply the two vectors by cross to obtain their common perpendicular vector N=(x,y,z), select points A and B (arbitrary) on the two lines respectively, and obtain vector AB. The projection of vector AB in the direction of vector N is the distance between the two non-coplanar lines (that is, the shortest distance). Do you know how to calculate it?
d=|vector N*vector AB|/|vector N| (the above is the scalar product of two vectors, the following is the modulus), let the intersection points be C and D, and substitute them into the symmetric formula of the common perpendicular line N. Since the two points C and D satisfy the initial straight line equation, we get two continuous equations about C (or D), and we can solve them separately.
Formula: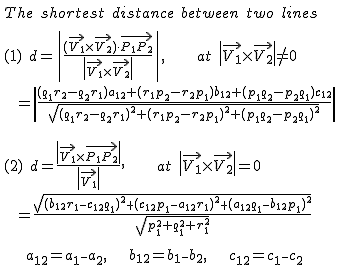
A Shortest Distance Between Two Lines Calculator is a tool that calculates the perpendicular distance between two lines in space (either 2D or 3D). The shortest distance is the minimum distance between the two lines, measured along the line that is perpendicular to both of them.
Why Use a Shortest Distance Between Two Lines Calculator?
The shortest distance between two lines is a useful concept in geometry and physics. It's particularly important when working with parallel or skew lines in 3D space, as the shortest distance represents the most direct path between them.
When to Use a Shortest Distance Between Two Lines Calculator?
You would use this calculator in the following situations:
- Geometry problems: When asked to find the shortest distance between two lines, especially in 3D space.
- Physics problems: When calculating the distance between two paths, such as the trajectory of two moving objects.
- Engineering or design problems: When analyzing how far apart two linear structures or paths are.