Voltage across Inductance Calculator

Enter value and click on calculate. Result will be displayed.
Inductance:
Rate of change of current:
Time:
Result:
Voltage across Inductor:
---

A Voltage across Inductance Calculator is a tool used to calculate the voltage across an inductor in an electrical circuit. The voltage across an inductor is crucial in understanding how inductors affect the behavior of circuits, especially in alternating current (AC) or time-varying direct current (DC) circuits.

Why use a Voltage across Inductance Calculator?

  • Circuit analysis: When designing or analyzing circuits, understanding the voltage across an inductor helps in predicting the overall behavior of the circuit (such as resonance in AC circuits or energy storage in DC circuits).
  • Efficient power management: In power systems, inductors are often used for filtering, energy storage, and voltage regulation. Calculating the voltage helps ensure the components are operating within their limits.
  • Time-varying currents: Inductors store energy in their magnetic fields and resist changes in current. The voltage calculation helps understand how the current through an inductor changes over time and how much resistance it introduces to the circuit.

How does a Voltage across Inductance Calculator work?

The voltage across an inductor is related to the rate of change of the current through it, and the formula is derived from Faraday’s Law of Induction:

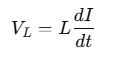
Where:

  • VL​ is the voltage across the inductor (in volts).
  • L is the inductance of the inductor (in henries, H).
  • dI​ / dt is the rate of change of current through the inductor (in amperes per second, A/s).

If the current I(t) through the inductor is given as a function of time, you can calculate dI​ / dt​ to find the voltage.

Example in a DC circuit:

For a simple RL circuit (resistor and inductor in series) with a current increasing linearly over time:

  • Suppose the current I(t)=2t (where tt t is time in seconds).
  • The inductance of the inductor is L=5H.

The voltage across the inductor at any time t would be:

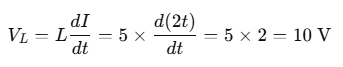
Thus, the voltage across the inductor is 10 volts.

Example in an AC circuit:

In AC circuits, the current typically varies sinusoidally, so:

  • I(t) = I0​ sin(ωt)
    • Where I0​​ is the maximum current and ω is the angular frequency.
  • The voltage across the inductor in this case is:

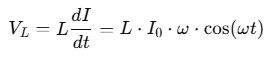
So, the voltage will also oscillate with the same frequency as the current but may have a phase difference.

When to use a Voltage across Inductance Calculator?

  • In RL circuits: When analyzing circuits that include resistors and inductors, either in DC or AC circuits.
  • In power electronics: To calculate how inductors affect voltage and current in transformers, motors, and other components.
  • For transient analysis: To understand how the voltage across an inductor changes as the current changes over time, especially during switching or transient events.
  • For energy storage applications: In circuits where inductors are used to store energy in their magnetic fields (e.g., in buck or boost converters, inductive charging systems).
  • When analyzing time-varying signals: Whenever the current in the circuit is changing with time, and you need to calculate how the voltage behaves as a result.