Doppler Effect Wavelength Behind Calculator
A Doppler Effect Wavelength Behind Calculator is a tool used to calculate the change in the wavelength of a wave when the observer is moving away from a wave source. In the context of the Doppler Effect, when the observer moves away from the source, the wavelengths of the waves are stretched, and the frequency decreases. This is the opposite of when the observer is moving towards the source (which compresses the wavelength).
What is the Doppler Effect Wavelength Behind?
The Doppler Effect refers to the change in frequency (or wavelength) of a wave in relation to an observer who is moving relative to the source of the wave. Specifically, when the observer is moving away from the source, the wavelength increases (the waves are stretched), and the frequency decreases. This effect occurs in all types of waves, such as sound, light, and electromagnetic waves.
For example, if you’re standing still and an ambulance with a siren is moving away from you, you’ll notice the sound of the siren decreases in pitch as the ambulance gets further away. This is because the sound waves are stretched, and the wavelength becomes longer, lowering the frequency.
Why use a Doppler Effect Wavelength Behind Calculator?
The Doppler Effect Wavelength Behind Calculator is useful for predicting the change in wavelength and frequency when the observer is moving away from the source. It is commonly used in:
- Radar and sonar systems: To calculate how the motion of a target away from the observer affects the wavelength of the signal.
- Astronomy: To study the redshift of light emitted by celestial objects moving away from Earth (e.g., distant galaxies).
- Communication systems: To model how moving receivers or transmitters affect the frequency of the signals.
- Acoustics: To understand how sound waves change when the source is moving away from an observer (e.g., a moving car with a horn).
How does a Doppler Effect Wavelength Behind Calculator work?
The Doppler Effect Wavelength Behind Calculator uses equations based on the relative motion between the observer and the wave source. The main goal is to calculate the change in wavelength (or frequency) when the observer moves away from the wave source.
For sound waves, the equation for the Doppler Effect when the observer is moving away from the source is:
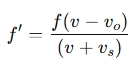
Where:
- f ′ = observed frequency
- f = emitted frequency (frequency of the source)
- v = speed of sound in the medium
- vo = speed of the observer (positive if moving away from the source)
- vs = speed of the source (positive if moving away from the observer)
For light waves, the relativistic Doppler effect formula is used:
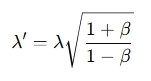
Where:
- λ′= observed wavelength
- λ = emitted wavelength
- β= v / c (the ratio of the velocity of the source to the speed of light)
In this case, the wavelength increases as the observer moves away from the source, and the frequency decreases. The observed wavelength λ′is larger than the emitted wavelength λ.
When to use a Doppler Effect Wavelength Behind Calculator?
A Doppler Effect Wavelength Behind Calculator is used when:
- The observer is moving away from the source: In situations where the observer is retreating or moving in the opposite direction of the source, causing the wavelength to stretch.
- Radar and sonar measurements: When determining the speed of objects moving away from the radar or sonar sensor, such as ships or airplanes.
- Astronomical analysis: To determine the redshift of light from celestial bodies that are receding from Earth, such as distant stars, galaxies, or quasars.
- Acoustic analysis: In situations where sound is emitted by a moving source, and the observer is moving away, such as cars, ambulances, or other moving vehicles.
- Communication systems: In satellite or mobile communications, where the receiver may be moving away from the source, and you need to account for frequency shifts.