Elastic Potential Energy Calculator

I want to calculate
Elastic coefficient(k) = N/m
Spring stretch length(x) = m
Elastic potential energy(U) = J
 

An Elastic Potential Energy Calculator is a tool used to calculate the potential energy stored in a stretched or compressed elastic object, such as a spring. The elastic potential energy is the energy stored due to the deformation of an elastic material when a force is applied to stretch or compress it.

Why is it important?

  • Understanding Energy Storage: Elastic potential energy helps us understand how energy is stored in systems like springs or rubber bands. This concept is used in many areas of physics and engineering, from mechanical systems to oscillating systems.
  • Engineering Applications: Elastic potential energy is crucial for designing devices like suspension systems, shock absorbers, and spring-loaded mechanisms. Understanding how energy is stored and released helps in optimizing the performance of these systems.
  • Conservation of Energy: It’s important in energy conservation and transformation, as elastic potential energy can be converted into kinetic energy or work done in various mechanical systems.

How is it calculated?

Elastic potential energy (Ep) stored in a stretched or compressed spring is calculated using the following formula derived from Hooke’s Law:

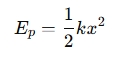
Where:

  • Ep​ is the elastic potential energy (in joules, J),
  • k is the spring constant (in N/m), which indicates the stiffness of the spring,
  • x is the displacement or compression/stretch distance from the equilibrium position (in meters, m).

This formula shows that the potential energy stored is directly proportional to the square of the displacement (how far the spring is stretched or compressed), and also depends on the stiffness of the spring.

When is it used?

An Elastic Potential Energy Calculator is used in various situations, including:

  • Mechanical Systems: To calculate energy stored in springs, shock absorbers, or suspension systems in cars, bicycles, or machinery.
  • Physics Problems: In problems involving oscillations, harmonic motion, or energy conversion, such as in simple harmonic oscillators (e.g., a mass-spring system).
  • Engineering Design: When designing systems that require energy storage and release, such as in spring-loaded mechanisms, trampolines, or even in certain types of toys.
  • Energy Conservation: In any system where elastic materials are used, understanding and calculating elastic potential energy helps in analyzing the energy flow and ensuring efficient use of energy.

In summary, an Elastic Potential Energy Calculator helps in determining the energy stored in an elastic material under deformation, which is vital for understanding how energy is managed and transformed in mechanical systems and engineering designs.